Introduction to hand reading, funnel theory and counting combinations

There are 1326 different starting hand combinations in No-Limit Hold’em. Without a system that would help us decrease this number of all possible combinations an opponent may hold based on all the information we have, it would be practically impossible to determine the most likely hand an opponent is holding.
 
Therefore we resort to the “funnel theory” in poker, which helps us decrease this 1326 different combinations to a number that can be helpful to make good decisions at the table.
 
The principle itself is relatively easy to understand, as it basically decreases the number of potential holdings of an opponent, based on:

• Position
• Action beforehand
• Cards on the board
• The information on the opponent itself and the tendencies of the general population

In the following scenario, we will demonstrate how to use the principle in praxis, as well as discuss counting combinations, blockers, and the basics of poker mathematics.

Example

The opponent is sitting in the BTN (button, dealer), and we know he is aggressive and a solid player.
 
Preflop
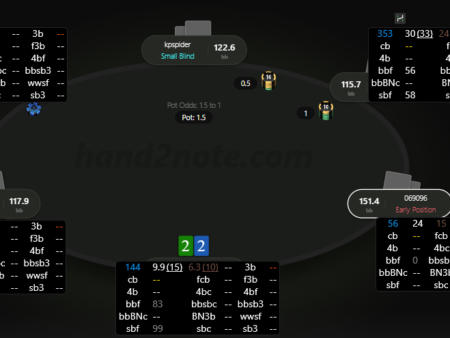
The opponent opens for 2.5bb and we 3-bet him from the Small Blind to 9bb, holding AcQc. The opponent calls our 3-bet.
 
Flop
Flop comes Ah6c7c, with 19bb in the pot. We make a c-bet of 30% (which equals 6bb), and the opponent calls
 
Turn
Turn comes the 6d, with the total pot being 31bb. Hero (us) bets 13bb, and the opponent calls.
 
River
River comes the 2d, with the total pot being 57bb. Hero bets 20bb, and the opponent goes all-in for a total of 72bb. We need to call 52bb for the total pot of 72bb+52bb+20bb, giving us 26% pot odds to call: 52/(72+52+20)=0,26.

The Funnel Theory in practice

Now we can try to make the best, educated decision using the principle:

• The normal opening range on the BTN is around 47% (22+, A2s+, K2s+, Q2s+, J2s+, T4s+, 94s+, 84s+ 73s+, 63s+, 53s+ 43s, A2o+, K8o+, Q9o+, J9o+, T9).
• Our 3-bet will be called with about 20% of that range ((99-22, AJs-A2s, KTs +, QTs +, JTs, T8s +, 97s +, 86s +, 75s +, 65s, 54s, AQo-ATo, KTo +, QTo +, JTo).
• On the flop he will call with about 60% of those hands: sets, two pair, top pair, second and third pair, open-ended draws, gutshots, and flush draws.

With this analysis, we already decreased the number of combinations to 9% of the starting hands (118 combinations).

• When we make another bet on the turn, we expect the opponent to fold the weakest holding in his range and continues with 66 combinations: two pair or better, flush draws, and strong open-ended straight draws.
• On the river, we make a bet to get value from weaker Ax hands, but the opponent shoves over our bet.

He is extremely polarized in this situation. We do not expect him to go all-in with Ax hands, but only with stronger combinations and bluffs, therefore his range looks something like this:
 
The opponent is shoving 13 combinations for value:

• Four of a kind: 1 combination
• Full House: 6 combinations
  77 only has 3 combinations with one 7 on the board. Only one combination of As6s and 2 combinations of 76s.
• Three of a kind: 6 combinations
  8h6h, 8s6s, 6s5h, 6s5s, 6h4h, 6s4s.

But with what can the opponent bluff?
 
Given the fact that we block Ax flush draws, he can have:

• Missed flush draws: 8 combinations
  KcJc, KcTc, JcTc, Kc9c, Tc9c, Tc8c, 9c8c, 5c4c.
• Missed straight draws: 3 combinations
  98s (we counted 9c8c already)

If he then really went all-in with all the missed draws, if we call, we will win 45% of the time.
Given the fact that our pot odds equal 26%, we can conclude we have a profitable call.

There would be slightly different results if our opponent would decide to turn some of his 7x and Ax hands into bluffs (there are reasons for such a move), and not bluff all the missed draws, and at the same time just call with some of his three of a kind combinations, but we will leave the more in-depth analysis for the future articles.
 
Counting combinations while playing is no easy task, but with practice, you can get really good at estimating these ranges while playing and significantly improve your game and results.